by Sanita Vetra-Carvalho
The second Maths Foresees study group was held on 3rd-6th April 2017, hosted by the Turing Gateway to Mathematics at the Isaac Newton Institute, Cambridge. The Maths Foresees network was established in May 2015 under the EPSRC Living with Environmental Change (LWEC) umbrella to forge strong links between researchers in the applied mathematics and environmental science communities and end-users of environmental research. The Maths Foresees events take a collaborative approach to industry problem solving where over the course of four days, mathematical and environmental scientists explored real challenges posed by companies operating in the environmental sector.
In this second event, there were five industry challenges presented to the participants (around 50 in total) from three companies: JBA, Sweco and Environmental Agency. All of the challenges this year were linked to flooding issues:
-
A Risk-based Analysis of Small Scale, Distributed, “Nature-based” Flood Risk Management Measures Deployed on River Networks (JBA Trust)
-
Modelling Sewer Networks (Sweco)
-
Understanding Rainfall Patterns (Environment Agency)
-
Estimating Flood Probability Using Historical Data (Environment Agency)
-
Making Decisions Using Uncertain Forecasts (Environment Agency)
I joined the group interested solving sewer modelling challenge proposed by Sweco and presented by James Franklin. The urban flood model InfoWorks ICM (Integrated Catchment Modeling) by Innovyze that is used by Sweco, comprises a subsurface sewer network and a street-level road surface model. The two are coupled via manholes but smaller drains/gullies are not included since the exact locations of gullies and drains are not known (it would be very costly in manpower to locate them) and more importantly it would be computationally unfeasible to directly model gullies in InfoWorks model. As a consequence, the model does not represent floodwater drainage correctly. In a typical simulation, floodwater stays on the road surface and does not drain away as it should. This results in an inaccurate flood extents, particularly in urban environments (see an image below of a typical simulation of a storm).

The challenge for the group was to see how we could improve the model representation of the collection network; that is how to represent gullies in the model to simulate a more realistic exchange (sinks and sources) of surface water between the sewer network and surface model.
Our group had two and half days to propose a solution. Our initial idea to couple a 2D surface shallow water model to a 1D sewer network model (also shallow water model) to model realistic fluid exchange between the two models turned out to be too difficult to accomplish in the limited time period. Hence, we concentrated our efforts on the main problem at hand, how to represent realistic sinks in the model without directly resolving gullies in the model. To this end, our group produced two 2D surface models: 2D shallow water model and 2D diffusive wave model. The second model was developed in parallel as in a future it would be easier to couple to a 1D drainage network. Our group run both models on an idealised road setting: 100m straight road with 3 manholes every 30m and 20 gullies every 10m, where directly resolved (see image below).
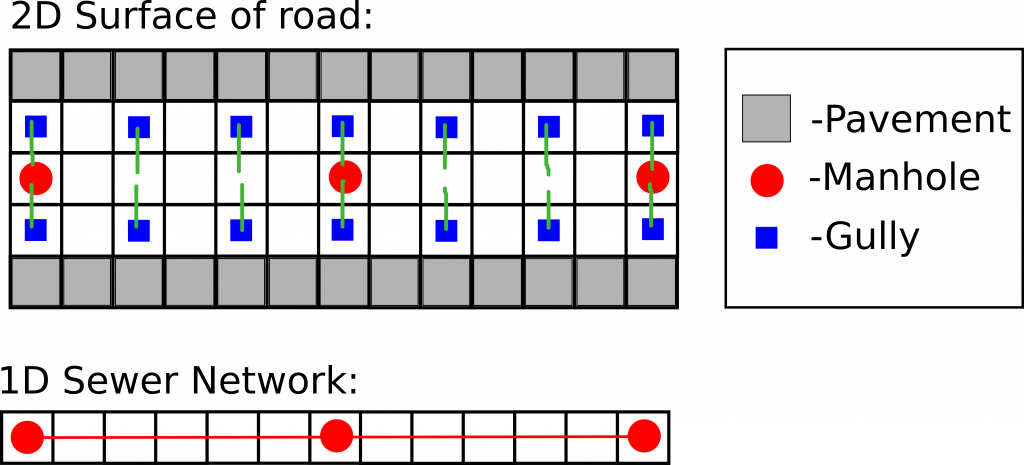
We compared runs where we resolved gullies directly on the mesh every 10m on both sides of the road (the case which is computationally unfeasible for Sweco to run but is the most realistic) to line sink runs where we averaged the effect of the number gullies on the road and removed the surface liquid from the model at each gridpoint that is adjacent to the pavement. Both of our 2D surface models showed that the line sink representation of the gullies removed approximately the same volume of surface water in the model as directly resolving each gully in the model thus making line sink solution a realistic and computationally affordable to represent the effect of gullies in the model. While our solution lacked the two-way flow exchange between the surface model and sewer network we proposed that if implemented in the InfoWorks model the volume of water sunk through line sinks would become a source in the sewer network through the nearest manhole in the model. Our findings and the proposed solution to the Sweco challenge was positively received by James Franklin. A full report of our solution will be published on Turing Gateway to Mathematics site over next two months.
I very much enjoyed being part of the Maths Foresees study group 2017 and am very thankful to all the organisers at MathsForesees network and Turing Gateway of Mathematics for organising this event as well as Isaac Newton Institute for hosting it. It was very refreshing to be ‘locked’ into the Isaac Newton Institute alongside other participants to solve these challenges in a mentally very rich and inspiring environment. The event naturally offered a very fruitful ground for networking too. I would encourage any mathematician interested in solving environmental problems to take a part in any future MathsForesees events!

